Error development in the glass literature > 

History, prospects, and problems of measurements and calculations of glass properties (PDF, 76 kB)

Oleg Mazurin

Thermex Company, St-Petersburg, Russia. E-mail: mazurin@itcwin.com

Presented at the Third Balkan Conference on Glass Science and Technology

26-30 September 2005, Varna. Bulgaria

Abstract: In the paper the survey of changes in specific features of publications of experimental data on glass properties is presented. The changes in the number of studied glass, and frequency of the studies of different glass properties and glass components are described and discussed. A steady decline of reliability of the published glass property data is demonstrated. The possibilities to determine the most reliable property values for binary and complex glass compositions by using a glass property database are described.

Keywords: glass property; reliability of property data; property calculations; history of property studies, SciGlass.

INTRODUCTION

The importance of comprehensive information on glass and melt properties both for the glass science and technology is obvious. It also seems obvious that the problem of reliability of glass property data is crucial for any use of such data. Very often a specialist on glass is interested in prediction of properties of glasses that have not been studied so far. In this case he/she should use the existing methods of property calculations. All these methods are also based on experimental property data. Thus, the existing amount of glass property data is the foundation for the whole building of the present-day glass science and technology.

To use correctly the existing glass property data and judiciously plan further studies some trends in the measurements of glass properties made during the 20^th and the beginning of the 21^st century should be studied. In the paper the ways to obtain reliable data by using the existing methods of glass property calculations are also described.

Such survey should be based on a good glass property database. At present the greatest amount of data (for 267,000 glasses) are compiled in the SciGlass database [1]. Thus this database was used to prepare all figures and tables in the present paper.

THE HISTORY AND TRENDS OF GLASS PROPERTY MEASUREMENTS

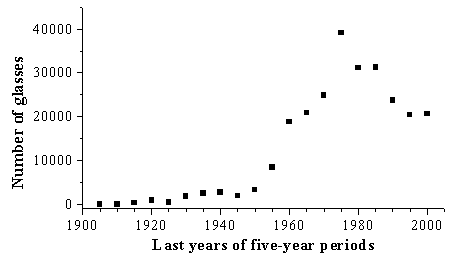
In Fig.1 the total number of glasses whose properties were published during the five-year periods of the 20^th century is presented. It is to be noted that although the authors of SciGlass have tried to compile all glass property data published worldwide, they inevitably have missed some portion of them. And yet the general dependence shown in the figure should be considered as a correct one because the percentage of missed data must be more or less the same for all periods of time.

Fig.1: Intensity of glass property measurements

A steady increase in the activity of glass property measurements began in the middle of the 20^th century and lasted until 1975. In the 80-ies a decrease in the scientific activity began. There is a possibility that in the last decade the number of studied glasses per year has stabilized. Estimations of these values in the period from 2001 to 2005 support this supposition.

It is desirable to analyze the history of glass property studies in several aspects. Table 1 shows the changes in the interests of scientists to the studies of some glass properties.

In the table the ratios of glasses, for which a property was measured during a ten-year period, to all studied glasses is presented. These ratios are given in percent. In the left column the last years of each period are indicated. Obviously the year of 2005 indicates the end of a five-year period. However owing to the presentation of relative characteristics in the table, the data shown in this last row are reliable enough.

In view of the special interest to the prospects of glass studies it is reasonable to pay attention only to the data obtained within the next few decades.

All properties presented in the table can be divided into three main groups: properties to which the interest of scientists remains more or less stable, properties, the interest to which is increasing, and properties, the interest to which is decreasing.

High-temperature viscosity, refractive index, micro-hardness, and chemical durability belong to the first group. All these properties are quite important for the glass science and technology. Density and Tg belong to the second group. The most remarkable is an intensive increase of interest of scientists to Tg measurements. At present most of such data are obtained by the Differential Scanning Calorimeter (DSC) method that requires minimum efforts of specialists. It is to be noted that scientists measuring Tg by the DSC method are divided now into two big groups. One group determines Tg by the center of a specific curvature on a DSC curve while the other one does it by the center of the endothermic minimum on a curve. The difference between these two values equals to 30-50 K. In addition, many authors even do not report the way Tg was determined. Thus at present this property appears to be the least important one. It seems obvious that the main appeal of this property for the authors is the measurement simplicity. Probably, this simplicity of the measurements is also the reason of a rapid increase in the percentage of density measurements.

For the last three properties presented in the table the general decline in the interest of scientists to such kinds of measurements can be stated. These properties are quite important both from the practical and theoretical points of view. Probably, the decline of interest  to such measurements results from the difficulties of the measurements of high-temperature density and surface tension, as well as from the time consuming procedure of the studies of glass-formation areas.

Table 2 shows the frequency of the studies of the glasses containing selected components.

The most interesting here are the data for Li₂O, SrO, and ZnO. In my view an impressive rise of interest to these components is an indication of an increase in the interest of the glass industry to the use of these components in commercial glasses.

It is essential to note the changes in the average number of authors of one paper. These changes are demonstrated by Table 3.

In my view it is a negative tendency. The excessive number of authors lead to a decrease in the feeling of responsibility of each of the authors.

However, the most important change in the characteristics of the papers containing glass property data is a gradual decline in reliability of the reported property data. This factor should attract attention of those who use glass property values in their work or make measurements of these data by themselves. That is why it is reasonable to discuss this problem in some detail.

In general, if you do not know the authors of a paper personally, it is impossible to judge whether the data presented in the paper are reliable or not. Thus you can only hope that the authors are qualified and responsible enough to publish really good property data. Unfortunately, often enough these hopes prove to be unfounded.

The only possibility in this case is the use of the widely applied idea of the Round Robin tests. If you sent the same glass to several laboratories and statistically processed the obtained measurement results you would have been able to obtain quite reliable property value. For us it is not necessary to send glass specimens to a number of laboratories. In many cases we possess the results of property measurements of glasses of the same system performed by quite a few authors. Processing of such data for binary glasses can be carried out by applying the SciGlass database. It is remarkable that when the number of sources is high enough the compiled points form a distribution around the most probable values that is approximated well enough by the Gaussian curve (see, for example, Ref. [2]).

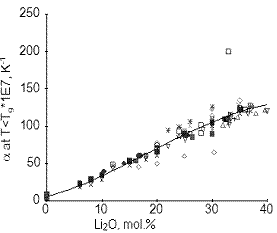
Fig.2: Thermal expansion coefficient of solid lithium silicate glasses

All you need is to form a corresponding query, plot the dependence of the property of interest on concentration and select the order of a polynomial. If the number of the sources containing the required data is great enough, you can be sure that the obtained dependence is near enough to the true one.  Fig.2 shows an example of such types of plots.

The solid line is an approximation of 180 points taken from more than 50 sources. Accordingly this dependence is absolutely reliable. Most of the experimental data are positioned in a reasonably close proximity of the approximating curve. There are a number of outliers, as well. The greatest errors have been found in two publications: three points well below the curve (Ref. [3]) and one point at a particularly great distance above the curve (Ref. [4]). Note that the latter paper has been published quite recently in such a respected journal as Phys. Chem. Glasses. In the paper the influence of Al₂O₃ on various properties of lithium silicate glasses was studied. The demonstrated result casts doubts on the correctness of any of the numerous data in this paper. It seems obvious that, if the referee of the journal used a glass property database, such paper would have never appeared in the journal pages.

Often enough not only the absolute values of the reported data are totally wrong, but also the character of composition dependencies. An example of this is demonstrated by Fig.3.

Curve 1 is a part of the approximating curve. The total curve was drawn by the least-squares method on the basis of 263 points taken from 79 different sources. Curve 2 is drawn on the basis of 5 points taken from Ref. [4]. Probably, no comments are needed here. Some other examples of false composition dependencies are given in Ref. [5].

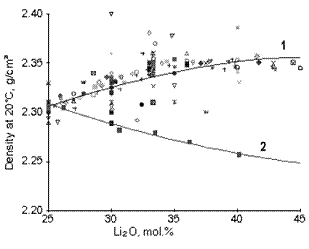
Fig.3: Density of binary lithium-silicate glasses

If you work with the SciGlass database, you will be able to see that obviously wrong data in recent publications have been found more often than in old ones. Fig.4 shows the results of statistical evaluation of the data describing the dependence of density on composition of sodium-silicate glasses (to obtain the approximated dependence 607 points taken from more than a hundred sources have been used).

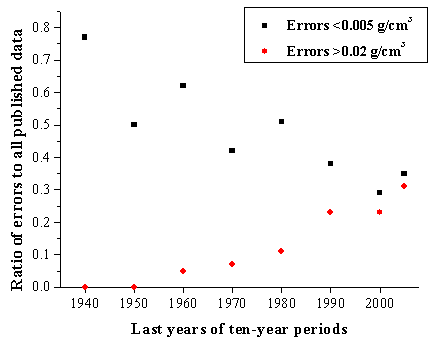
Fig.4: Quality of the experimental data on density of Na₂O-SiO₂ glasses

For each decade the numbers of the most precise (Errors<0.005 g/cm³) and most erroneous (Errors>0.02 g/cm³) results have been divided by the total numbers of density values published within the selected decade. The tendency of a gradual decrease in reliability of glass property data is obvious.

CALCULATIONS OF GLASS PROPERTIES

It is clear that for the binary glasses whose properties have been studied by a considerable number of scientists the statistical approximations of the type shown in Figs 2 and 3 is the best way to predict a property of any glass belonging to a selected system. However, for multi-component glasses it is necessary to use a somewhat different approach, namely, the application of the methods of property calculations. For most glass properties we can find from ten to twenty methods of property calculations in SciGlass. If we select a definite glass composition, the number of methods applicable to this composition proves to be smaller. Nevertheless, one can usually find quite a few methods that could be used for calculations. Accordingly, the problem of selection of the best method arises. As was demonstrated in Ref. [7], the changes in the composition areas often lead to the changes of the most reliable calculation methods. To find the most reliable method is possible only by comparing the results of property calculations by all available methods with experimental data for glasses belonging to a system of interest. Next the method with a minimal root-mean-square error is selected and this method is used to calculate the required value. At present it is the best way to obtain a property value that is as near to the true one, as possible.

Let us find the best method to calculate T₃ in commercial glasses of a soda-lime-silica group. To search the experimental data (in mol%) we have selected the following composition area : Al₂O₃: 0 - 2; MgO: 0 - 8; SiO₂: 60 - 80; Na₂O: 10 - 20; CaO: 5 - 15. 143 points have been found, they have been taken from 50 different sources of information.

The following methods of calculation can be used to calculate T₃ for all or nearly all (minus one or two points) selected compositions: the ones by Lakatos, Lyon, Mazurin et al., Priven-98, Priven-2000, and Shaw. The descriptions of these methods and the corresponding references can be found in Ref.[1]. At first the mean values of the results obtained by all six methods have been calculated and compared with the experimental ones. By such a comparison seven publications with particularly great deviations of experimental data from the calculated ones have been determined and their data have been excluded from further calculations. Thereafter the following characteristics of the applied calculation methods have been obtained (see Table 4):

In the row "Shift" the systematic differences between the calculated and experimental values are shown. The random error is the root-mean-square differences between the calculated and experimental errors after subtraction of the "Shift" values from these differences. In the "Sum" row the square roots of the sum of the squared "Shift" and "Random" values are shown.

It is seen from the table that in our case the most reliable methods are those by Lakatos and Lyon. It is reasonable to find for each composition the mean values of the calculation results obtained by both these methods. In general it should lead to an additional decrease of errors. The root-mean-square error of the calculations by these two methods is lower than 15 K, i.e. less than 0.1 difference on the log₁₀ viscosity scale. For most of the practical applications it is quite a reasonable error.

CONCLUSION

In the studies of glass properties and the use of the results of these studies we can see several general trends that have appeared during the last few decades. They are as follows.

1. The number of publications per year has reached the maximum in the period of 1970-1975 having decreased quite considerably thereafter.
2. The frequency of the measurements of easily determined properties increases while the frequency of some quite important but labor-consuming property measurements decreases steadily.
3. The average number of authors per paper increases.
4. The percentage of papers with erroneous data rises steadily.

REFERENCES

1. SciGlass-6.5. Institute of Theoretical Chemistry, Shrewsbury, MA, 2005.
2. O. Mazurin and Yu. Gankin: "About testing the reliability of glass property data in binary systems"; J. Non-Cryst. Solids 342 (2004) 166-169.
3. H. El-Batal, N. Ghoneim, A. Ahmed, and F. Khalifa. Centr. Glass Ceram. Res. Inst. Bull. 25 (1978) 99-110.
4. G. El-Damrawi and H. Doweidar. Phys.Chem.Glasses 42 (2001) 116-120.
5. V. Rajendran, F. Khaliafa, and H. El-Batal. Indian J.Phys. 69A (1995) 237-242.
6. O. Mazurin: "Glass properties: compilation, evaluation, and prediction"; Non-Cryst. Solids 351 (2005) 1103-1112.
7. A. Priven and O. Mazurin: "Comparison of methods used for the calculation of density, refractive index and thermal expansion of oxide glasses"; Glass Technology 44 (2003) 156-166.